【LetMeFly】1976.到达目的地的方案数:单源最短路的Dijkstra算法
力扣题目链接:https://leetcode.cn/problems/number-of-ways-to-arrive-at-destination/
你在一个城市里,城市由 n 个路口组成,路口编号为 0 到 n - 1 ,某些路口之间有 双向 道路。输入保证你可以从任意路口出发到达其他任意路口,且任意两个路口之间最多有一条路。
给你一个整数 n 和二维整数数组 roads ,其中 roads[i] = [ui, vi, timei] 表示在路口 ui 和 vi 之间有一条需要花费 timei 时间才能通过的道路。你想知道花费 最少时间 从路口 0 出发到达路口 n - 1 的方案数。
请返回花费 最少时间 到达目的地的 路径数目 。由于答案可能很大,将结果对 109 + 7 取余 后返回。
示例 1:
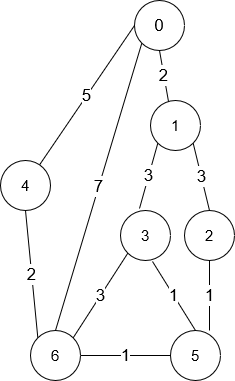
输入:n = 7, roads = [[0,6,7],[0,1,2],[1,2,3],[1,3,3],[6,3,3],[3,5,1],[6,5,1],[2,5,1],[0,4,5],[4,6,2]]
输出:4
解释:从路口 0 出发到路口 6 花费的最少时间是 7 分钟。
四条花费 7 分钟的路径分别为:
- 0 ➝ 6
- 0 ➝ 4 ➝ 6
- 0 ➝ 1 ➝ 2 ➝ 5 ➝ 6
- 0 ➝ 1 ➝ 3 ➝ 5 ➝ 6
示例 2:
输入:n = 2, roads = [[1,0,10]]
输出:1
解释:只有一条从路口 0 到路口 1 的路,花费 10 分钟。
提示:
1 <= n <= 200n - 1 <= roads.length <= n * (n - 1) / 2roads[i].length == 30 <= ui, vi <= n - 11 <= timei <= 109ui != vi- 任意两个路口之间至多有一条路。
- 从任意路口出发,你能够到达其他任意路口。
方法一:单源最短路的Dijkstra算法
“单源最短路”意思是从一个点出发到其他点的最短路径。单源最短路的Dijkstra算法也可以看我之前做的视频。
总之Dijkstra算法就是,我们从起点开始:
计算所有能一步到达的点中,哪个点距离起点最近。
下一步就走到这个点,然后能一步到达的点就更新了。
直到走完所有的点为止。
对于这道题,我们在“往前走”的同时,记录一下走到这一步的“方案数”:
- 若从当前点走到点
a的距离 小于 a原本到起点的距离,则说明发现了新大“路”(更近的路)。舍弃掉之前的方案数,将点a的方案数变为当前点的方案数,并更新最短距离,可以从点a开始往深处继续探索。
- 若从当前点走到点
a的距离 等于 a原本到起点的距离,则说明又发现了一条同为最近路的路。将点a的方案数加上当前点的方案数。
- 否则,已有更短路,不做考虑。
最终返回终点的路径数即为答案。
- 时间复杂度$O(m\log m)$
- 空间复杂度$O(n+m)$
AC代码
C++
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
| typedef long long ll;
const ll MOD = 1e9 + 7;
class Solution {
public:
int countPaths(int n, vector<vector<int>>& roads) {
vector<vector<pair<int, int>>> graph(n);
for (vector<int>& road : roads) {
graph[road[0]].push_back({road[1], road[2]});
graph[road[1]].push_back({road[0], road[2]});
}
vector<ll> way(n);
way[0] = 1;
vector<ll> dis(n, 1e18);
dis[0] = 0;
priority_queue<pair<ll, int>, vector<pair<ll, int>>, greater<pair<ll, int>>> pq;
pq.push({0, 0});
while (pq.size()) {
auto [thisDistance, thisNode] = pq.top();
pq.pop();
if (thisDistance > dis[thisNode]) {
continue;
}
for (auto [nextNode, nextDistance] : graph[thisNode]) {
if (thisDistance + nextDistance < dis[nextNode]) {
dis[nextNode] = thisDistance + nextDistance;
way[nextNode] = way[thisNode];
pq.push({dis[nextNode], nextNode});
}
else if (thisDistance + nextDistance == dis[nextNode]) {
way[nextNode] = (way[nextNode] + way[thisNode]) % MOD;
}
}
}
return way.back();
}
};
|
Python
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
|
MOD = int(1e9) + 7
class Solution:
def countPaths(self, n: int, roads: List[List[int]]) -> int:
graph = [[] for _ in range(n)]
for x, y, d in roads:
graph[x].append((y, d))
graph[y].append((x, d))
way = [0] * n
way[0] = 1
dis = [int(1e18)] * n
dis[0] = 0
pq = [(0, 0)]
while pq:
thisDistance, thisNode = heapq.heappop(pq)
if thisDistance > dis[thisNode]:
continue
for nextNode, nextDistance in graph[thisNode]:
if nextDistance + thisDistance < dis[nextNode]:
dis[nextNode] = nextDistance + thisDistance
way[nextNode] = way[thisNode]
heapq.heappush(pq, (dis[nextNode], nextNode))
elif nextDistance + thisDistance == dis[nextNode]:
way[nextNode] = (way[nextNode] + way[thisNode]) % MOD
return way[-1]
|
同步发文于CSDN和我的个人博客,原创不易,转载经作者同意后请附上原文链接哦~
Tisfy:https://letmefly.blog.csdn.net/article/details/136481215
